Tag: Pure Data

Posted on

Creating Polymetric Beats Using Pure Data

I am giving a presentation at Synthfest on November 9th, 2024 in Burlington, Massachusetts. This workshop is related to my ongoing research in using Pure Data as a tool for computer-assisted composition, sound processing, and sound synthesis. Specifically, the presentation is on how to use Pure Data to create polymetric beats. A hackable template is available below for download. You can hear such beats in my most recent album, Rotate (Bandcamp, Spotify, Apple, Amazon).

drumpoly_shell.pdDownload

Pure Data is often used as a tool for sound synthesis or signal processing. A quick history lesson reminds us however that it is also a robust tool for algorithmic, or computer-assisted composition. Pure Data is an open source visual programming environment for sound and multimedia. It is strongly based upon the programming environment Max. When Max first added the ability to process and generate audio and video, it was referred to as Max/MSP/Jitter to highlight these new abilities. However, going back to the very beginnings of Max, originally developed at IRCAM in 1985 by Miller Puckette (who also developed Pure Data), it was centered on interactive, algorithmic and computer-assisted composition.

Defining the Problem

In order to create polymetric beats in Pure Data, it is useful to think of two steps in the process. The first is how do we represent musical patterns in a way that is easy to codify for computers. The second is how do we read that pattern in such a way that we can fire off a MIDI note at the correct time to realize that pattern.

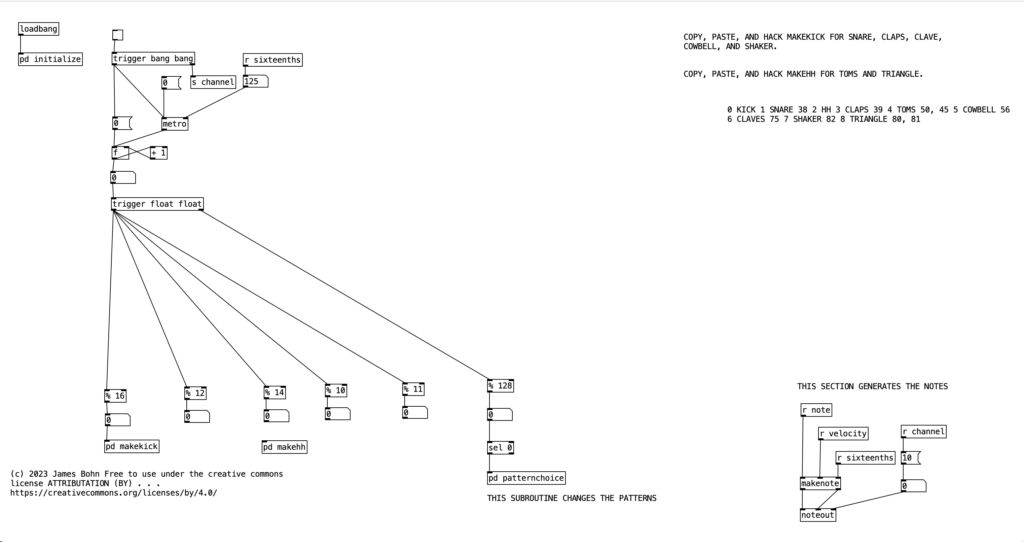
Attached to this blog post is a program shell that we will use to understand a basic process where patterns are defined and initialized, a time structure, a structure for evaluating whether a note should happen, an algorithm for firing off a note, and a structure for changing musical patterns. The way this program shell is designed, it currently only generates kick drum parts in common time. However, once we learn how this algorithm works, we can hack it to create far more complicated beats.

Defining a Pattern

Generating rhythmic content using an algorithm is a very useful exercise, as we do not have to worry about pitch material. On the most basic level, we could think of a measure of as being a list of sixteenth notes (or whatever the fastest pulse of the desired rhythm is), and we can express the rhythm by using zeros where notes do not happen, and ones where notes are played. Thus, if we want to define a four on the floor kick drum part in common time, we could express it as . . .

1 0 0 0 1 0 0 0 1 0 0 0 1 0 0 0

When we define tables in Pure Data, the first number indicates where in the table we’re putting the values. Typically, we would want to start at the beginning of the table, which would be position 0. Thus, from now on in, I will start every rhythm description with a 0, resulting in . . .

0 1 0 0 0 1 0 0 0 1 0 0 0 1 0 0 0

This is pretty basic. If we wanted to go a bit more advanced, we could imagine two different options, a normal kick drum, which we’ll represent with the number 1, and an accented kick drum, which we’ll represent with the number 2. If we want to accent beat one of the measure, we now get the table description . . .

0 2 0 0 0 1 0 0 0 1 0 0 0 1 0 0 0

Notice, it would be pretty easy at this point to use the number zero for when notes do not occur, but use the MIDI velocity number(1-127) to indicate when a note should occur. Doing this, you can get very finely tuned dynamic drum parts. However, such subtlety is not for me, I’m more of a boom / bap / boom-boom / bap guy myself, so I will be sticking with accented and unaccented notes. Let’s look at this table definition as it occurs in the program shell. To get there, double click on the object labeled pd initialize, which is below loadbang . . .

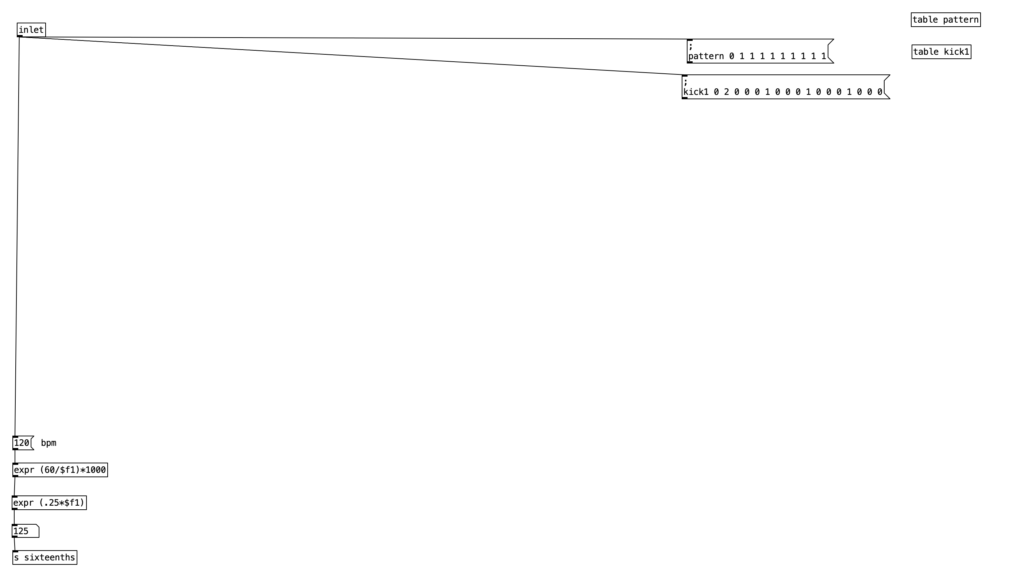
For those who are relatively new to Pure Data, loadbang designates algorithms that execute when you open the program. Likewise, any object in Pure Data that starts with the letters pd are subroutines. Double clicking them allows you to view and edit the algorithm contained inside the subroutine. Subroutines are a great way to declutter your screen, and to create algorithms that you may want to copy and paste into other programs you create. Note that the subroutine pd initialize also sets the tempo, translates that into milliseconds, and then into sixteenth notes (still expressed in milliseconds). Finally, we also see that pd initialize contains a definition for a table called phrase, but we’ll get to that later.

Changing Patterns

Before we get to how to use this kick drum pattern, I want to introduce one more level of complexity. In the long term it would be useful to define several different kick drum patterns so we can change patterns every eight measures or so to have a dynamic drum part. As we start to add more layers to the drum part (snare, high hat, toms, etc.) it would also be nice if some of those layers occasionally did not play at all, so the combination of layers also change as we move from phrase to phrase.

For the purposes of this algorithm, I’m going to allow for each layer of the drum part to select between three patterns (1, 2, & 3). Furthermore, I’ll also include a fourth possibility of 0, which would correspond to no pattern playing. In order to see how this is implemented in our algorithm, let’s look at the counter mechanism beneath the main metronome object. Beneath this metronome we see a trigger object, and beneath that, we see an object that states % 128. This is modular mathematics, which limits the value to a number between 0 and 127 (inclusive). We then feed the outcome of this to an object that states sel 0. When this object receives a 0 in its leftmost inlet, it will send a bang to its leftmost outlet. We then send this bang to trigger the subroutine pd patternchoice. Musically, what this means, is that the subroutine pd patternchoice executes at the beginning of every eight measure phrase. Since we are thinking in terms of sixteenth notes (8 x 16 = 128), a 0 would indicate the beginning of a phrase.

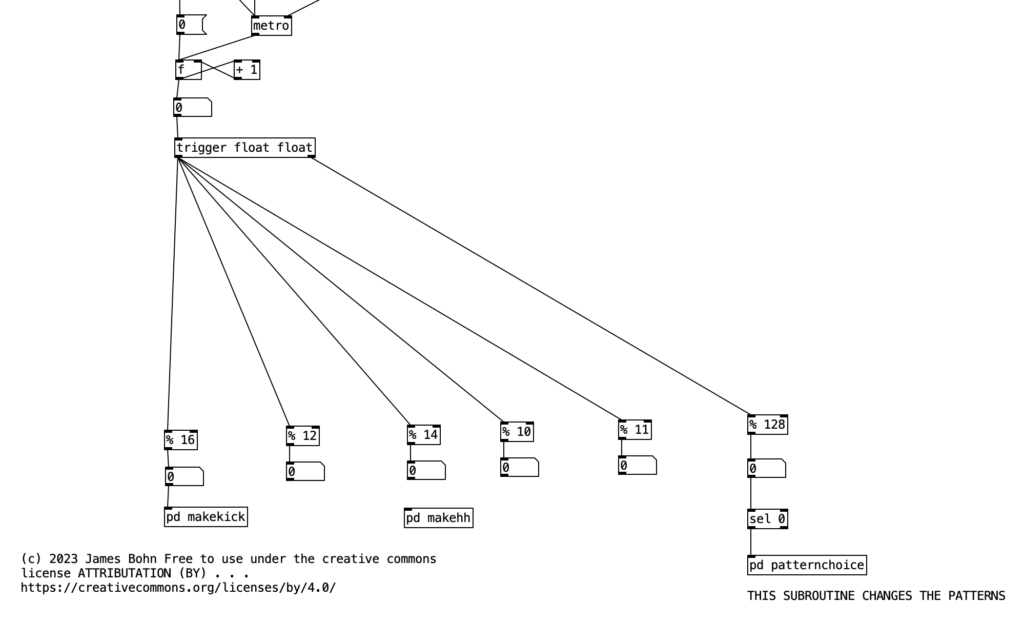
If we look inside pd patternchoice, we see two structures that are currently not connected to the inlet. In time we will copy and paste these structures, changing some of the numeric values, and connect them to the inlet. We will make these changes as we add more layers and patterns to our drum beat. However, for the time being since we only have one kick drum pattern, neither structure is connected to the inlet.

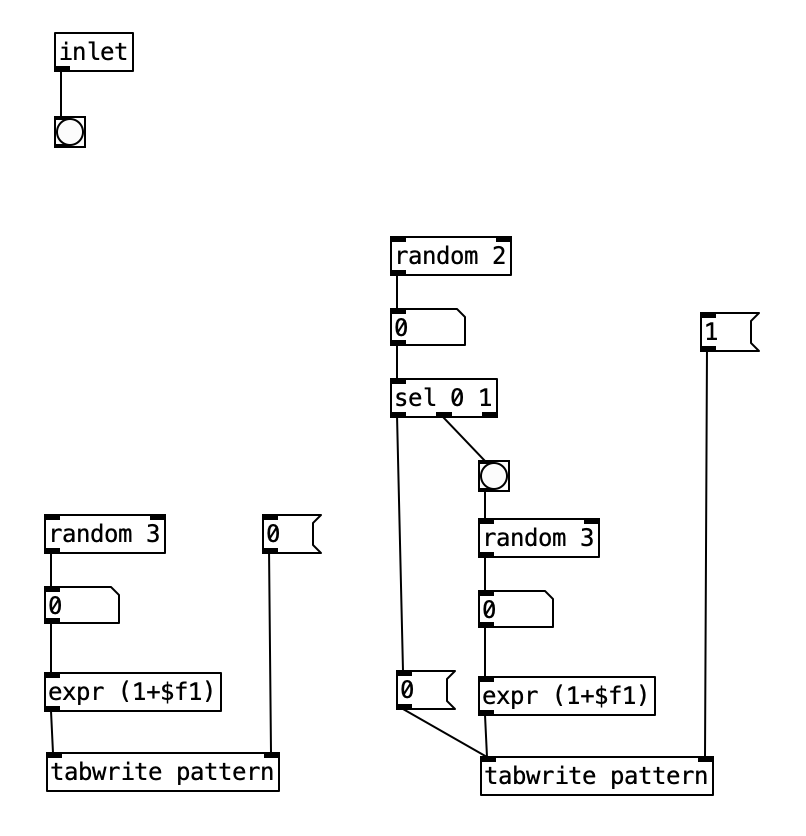
We will treat the algorithm on the left as being connected to the kick drum, while the algorithm on the right will eventually be used for the snare drum. Notice there is a difference between the two structures. The one on the left is simpler, and if we follow what it does, we figure out that that algorithm will write a 1, 2, or 3, but not a 0 to the table pattern in position 0. The structure on the right however, will write a 0 to the table patttern at position 1 half of the time. To put this into musical terms, the difference between the two means that kick drum will always be playing a pattern, while the snare drum will only play a pattern 50% of the time.

Firing Off a Note

Now lets see how pattern and kick1 are used to either fire off notes or not. We see at the bottom of the screen a makenote object connected to a noteout object that performs the final task of sending a midi note out. However pd makekick is the subroutine that determines whether or not a kick drum should occur at a given point. Above pd makekick we see the object % 16 which comes from the counter. This object mods the current counter to a number between 0 and 15 inclusive. These values correspond to the number of sixteenth notes in a measure of common time. Thus, when we return to the number 0 after the number 15 occurs it corresponds to returning to the beginning of the measure.

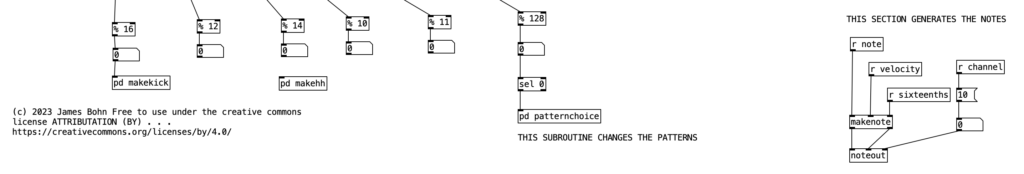
When we look at the structure pd makekick a lot of the heavy lifting of the subroutine is handled by the object spigot. Spigot receives numeric values through its leftmost inlet. However, it only passes those values through to its outlet if the numeric value being fed to its right most inlet is not zero. Thus, we can think of spigot as a valve that shuts off the flow of numbers when the rightmost inlet is zero. We can use this to selectively shut down the rest of the algorithm, which will effectively stop it from making notes.

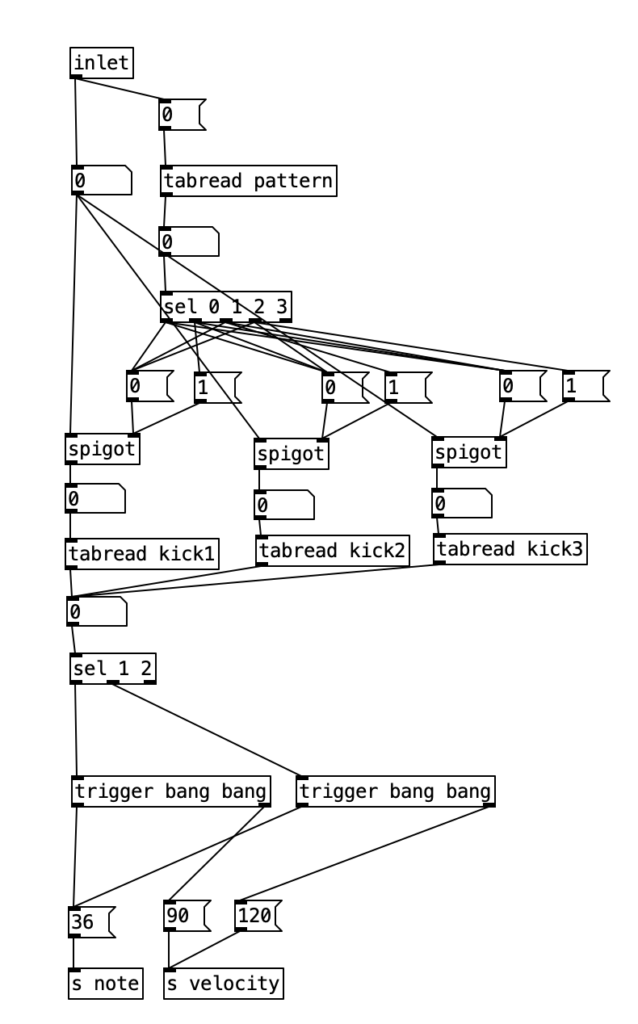
The first step is to determine whether a pattern should be playing or not. Likewise, at the same time we can determine which patterns should be playing if one occurs. To do this, we’ll read the value of pattern at array position 0 (which we’ll use to store the current kick drum pattern). We can then route the output of that to a number of different outcomes using a sel statement. Each outcome of the sel statement sends either a 0 or a 1 to the rightmost inlet of three spigots. These spigots correspond to the three possible kick drum patterns. Thus, when the pattern is 0, a 0 is sent to all three spigots, effectively shutting off the rest of the subroutine. When the pattern is 1, we send a 1 to the spigot for pattern one (turning it on), and a zero to the other two, making sure any previously used pattern is turned off, and so fourth.

Once we pass through one of the spigots, we encounter the part of the subroutine that determines whether a note should be fired at any given time. Underneath each spigot is a tabread object that reads the current position of a given pattern (kick1, kick2, or kick3, respectively). This will return the result of a 0, 1, or 2, corresponding to no note, normal note, and accented note. Since we don’t have to do anything when no note is played, we can simply ignore that result. All we have to do is correctly route the results for 1 and 2. Since all three patterns will be outputing 0, 1, and 2, we will treat those three results the same, we can route the output of each tabread statement to the same number box, and then route the results using sel 1 2. Both results will sending the number 36 to s note (36 is the MIDI note number corresponding to a kick drum), and will send a velocity of either 90 or 120 to s velocity.

Understanding Execution Order

In order to understand the mechanics of this, we have to understand a little about execution order for Pure Data. When an outlet branches off in several directions, Pure Data first executes the connection that was created first, regardless of where it is placed on the screen (in Max, it executes from right to left). When an algorithm branches off in this way, it travels all the way down until it hits the end, and then Pure Data goes back and executes the other branch of the algorithm. When an object has multiple outlets, it typically executes the rightmost outlet first (following it all the way down the algorithm) before it most to the next outlet.

Alternately, when an object has several inlets, the object typically does not spring into action until the leftmost inlet is triggered. We can think of new values that are connected to inlets that are note the leftmost inlet as queueing until the leftmost inlet is triggered. This order of execution in Pure Data allows non-crossed connections to fire in the correct order. To illustrate, imagine an object with two outlets and an object below with two inlets (similar to the makenote / noteout algorithm below). Furthermore, let’s imagine that the right outlet is connected to the right inlet of the object below, and the left outlet is connected to the left inlet of the object below. First, the value from the right outlet will queue at the right inlet of the object below, then the left outlet will send its value to the left inlet, triggering the object below.

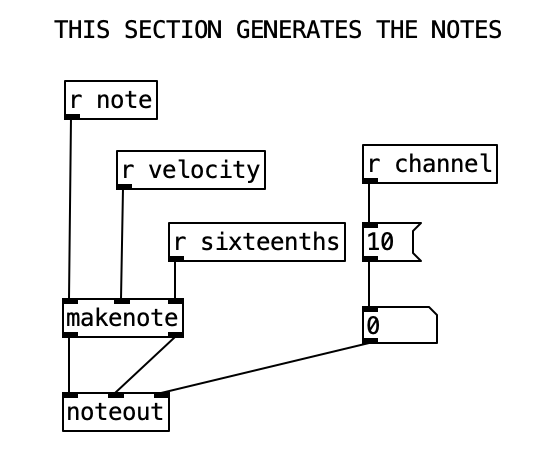
However, whenever we start to make complicated algorithms, it can be confusing to see in what order the algorithm will flow. When we want to force a specific order to achieve a specific outcome, or simply to make order clear, we can use a trigger object. A trigger takes a single inlet, and uses it to trigger several outlets (including the option of passing the inlet to one or more outlets) in a specific order. That order is (you guessed it, right to left). We will use this to control the order of sending the velocity and sending the note number.

The makenote object has three inlets that correspond to midi note number, velocity, and duration expressed in milliseconds. Since midi note number is the leftmost inlet, we have to send that value last. This means we have to send the velocity before we send the note number. Here we do not have to worry about duration, since duration is often irrelevant when triggering drum samples. Thus, we can set a duration of a sixteenth note during the initialization process.

Hacking the Shell

With the knowledge we have gained thus far, we are now equipped to start hacking the program shell. A good place to start would be to double click [pd initialize], copy both the object [table kick1] and the corresponding message that populates that table. You can then paste these objects, move them so they don’t overlap, change both to say kick2 instead of kick1, and change the numbers in the in the kick2 message to be a different pattern. Again, your pattern should only use the numbers 0, 1, and 2. Likewise, the table should be 17 numbers long with the first number being 0, in order to denote that we’ll load the array starting at the beginning. You can then connect the kick2 message to the [inlet] object.

Now do the same process again creating a table and message for kick3, making sure to connect the kick3 message to inlet. Now, we can finally make use of the subroutine [pd patternchoice] in the main algorithm. Double click on [pd patternchoice], and connect the outlet of the bang to both the [random 3] object and the message that contains the number 0. Now, when we run the the algorithm, we should hear the kick drum pattern randomly change once every eight measures.

Now we can get into the good stuff. Let’s add a snare drum part. First, we should decide what meter we want to use for the snare drum. For the purposes of this demonstration, let’s put the snare drum in three. We’ll start by copying the object [pd makekick] and pasting it. More the copied object under the [% 12] object, and connect the outlet of [% 12] to the inlet of the copied object. Let’s also rename the copied object to [pd makesnare].

We have to change some details of [pd makesnare] to get it to make a snare part. Double click the object and change the message box near the top from 0 to 1. Change the array names in the three [tabread] objects to be snare1, snare2, and snare3. Finally, right above the object [s note], change the number in the message box from 36 to 38 (the MIDI note number for snare drum).

Now, double click the object [initialize], and copy the three kick drum tables, as well as the three messages that define those tables. Paste those items, and move them so they don’t overlap with other items. change the array names to snare1, snare2, and snare3 in both the table objects and the messages that define the arrays. Now, let’s change the patterns. Since these are patterns that are in three, they will feature 12 sixteenth notes. Thus, each message will have to include 13 numbers, the first being the number 0 to indicate that the pattern is loaded at the beginning of the array. Again, use 0 to indicate no note, 1 to indicate a note, and 2 to represent an accented note. Make sure you connect each of the three messages to the inlet.

Now we need to allow the snare patterns to change, so let’s double click the subroutine [pd patternchoice]. In this subroutine we need to connect the outlet of the bang to both the [random 2] object and the message that contains the number 1. The [random 2] here yields a 50% chance that the snare appear in a given phrase. The [random 3] below that will select one of the three snare patterns when it is determined that the snare should appear in a phrase.

Posted on

Experiment 12: MBTA

As I mentioned in my previous experiment, it has been a busy and difficult semester for me for family reasons. Accordingly, I am two and a half months behind schedule delivering my 12th and final experiment for this grant cycle. Additionally, I feel like my work for the past few months on this process has been a bit underwhelming, but unfortunately this work what my current bandwidth allows for. I hope to make up for it in the next year or so.

Anyway, the experiment for this month is similar to the one done for experiment 11. However, in this experiment I am generate vector images that reference maps of Boston’s subway system (the MBTA). Due to the complexity of the MBTA system I’ve created four different algorithms, reducing the visual data at any time to one quadrant of the map, thus, the individual programs are called: MBTA NW, MBTA NE, MBTA SE, & MBTA SW.

Since all four algorithms are basically the same, I’ll use MBTA – NE as an example. For each example Knob 5 was used for the background color. There were far more attributes I wanted to control than knobs I had at my disposal, so I decided to link them together. Thus, for MBTA – NE knob 1 controls red line attributes, knob 2 controls the blue and orange line attributes, knob 3 controls the green line attributes, and knob 4 controls the silver line attributes. Each of the four programs assigns the knobs to different combinations of colored lines based upon the complexity of the MBTA map in that quadrant.

The attributes that knobs 1-4 control include: line width, scale (amount of wiggle), color, and number of superimposed lines. The line width ranges from one to ten pixels, and is inversely proportional to the number of superimposed lines which ranges from on to eight. Thus, the more lines there are, the thinner they are. The scale, or amount of wiggle is proportional to the line width, that is the thicker the lines, the more they can wiggle. Finally, color is defined using RGB numbers. In each case, only one value (the red, the green, or the blue) changes with the knob values. The amount of change is a twenty point range centered around the optimal value. We can see this implemented below in the initialization portion of the program.

	RElinewidth = int (1+(etc.knob1)*10)
	BOlinewidth = int (1+(etc.knob2)*10)
	GRlinewidth = int (1+(etc.knob3)*10)
	SIlinewidth = int (1+(etc.knob4)*10)
	etc.color_picker_bg(etc.knob5)
	REscale=(55-(50*(etc.knob1)))
	BOscale=(55-(50*(etc.knob2)))
	GRscale=(55-(50*(etc.knob3)))
	SIscale=(55-(50*(etc.knob4)))
	thered=int (89+(10*(etc.knob1)))
	redcolor=pygame.Color(thered,0,0)
	theorange=int (40+(20*(etc.knob2)))
	orangecolor=pygame.Color(99,theorange,0)
	theblue=int (80+(20*(etc.knob2)))
	bluecolor=pygame.Color(0,0,theblue)
	thegreen=int (79+(20*(etc.knob3)))
	greencolor=pygame.Color(0,thegreen,0)
	thesilver=int (46+(20*(etc.knob4)))
	silvercolor=pygame.Color(50,53,thesilver)
	j=int (9-(1+(7*etc.knob1)))

The value j stands for the number of superimposed lines. This then transitions into the first of four loops, one for each of the groups of lines. Below we see the code for red line portion of program. The other three loops are fairly much the same, but are much longer due to the complexity of the MBTA map. An X and a Y coordinate are set inside this loop for every point that will be used. REscale is multiplied by a value from etc.audio_in which is divided by 33000 in order to change that audio level into a decimal ranging from 0 to 1 (more or less). This scales the value of REscale down to a smaller value, which is added to the numeric value. It is worth noting that because audio values can be negative, the numeric value is at the center of potential outcomes. Scaling the index number of etc.audio_in by (i*11), (i*11)+1, (i*11)+2, & (i*11)+3 lends a suitable variety of wiggles for each instance of a line.

	j=int (9-(1+(7*etc.knob1)))
	for i in range(j):
		AX=int (320+(REscale*(etc.audio_in[(i*11)]/33000)))
		AY=int (160+(REscale*(etc.audio_in[(i*11)+1]/33000)))
		BX=int (860+(REscale*(etc.audio_in[(i*11)+2]/33000)))
		BY=int (720+(REscale*(etc.audio_in[(i*11)+3]/33000)))
		pygame.draw.line(screen, redcolor, (AX,AY), (BX, BY), RElinewidth)

I arbitrarily limited each program to 26 points (one for each letter of the alphabet). This really causes the vector graphic to be an abstraction of the MBTA map. The silver line in particular gets quite complicated, so I’m never really able to fully represent it. That being said, I think that anyone familiar with Boston’s subway system would recognize it if the similarity was pointed out to them. I also imagine any daily commuter on the MBTA would probably recognize the patterns in fairly short order. However, in watching my own video, which uses music generated by a PureData algorithm that will be used to write a track for my next album, I noticed that the green line in the MBTA – NE and MBTA – SW needs some correction.

The EYESY has been fully incorporated into my live performance routine as Darth Presley. You can see below a performance at the FriYay series at the New Bedford Art Museum. You’ll note that the projection is the Random Lines algorithm that I wrote. Likewise graduating senior Edison Roberts used the EYESY for his capstone performance as the band Geepers! You’ll see a photo of him below with a projection using the Random Concentric Circles algorithm that I wrote. I definitely have more ideas of how to use the EYESY in live performance. In fact, others have started to use ChatGPT to create EYESY algorithms.

Ultimately my work on this grant project has been fruitful. To date the algorithms I’ve written for the Organelle and EYESY have been circulated pretty well on Patchstorage.com (clearly the Organelle is the more popular format of the two) . . .

2opFM (Organelle) 2 likes, 586 views, 107 downloads
Additive Odd / Even (Organelle) 6 likes, 969 views, 184 downloads
Bass Harmonica (Organelle) 7 likes, 825 views, 174 downloads
Basic Circle (EYESY) 307 views, 7 downloads
Wavetable Sampler (Organelle) 2 likes, 796 views, 123 downloads
Basic Circles (EYESY) 1 like, 279 views, 16 downloads
Random Lines (EYESY) 198 views, 18 downloads
Random Concentric Circles (EYESY) 132 views, 18 downloads
Colored Rectangles (EYESY) 1 like, 149 views, 31 downloads
Random Rectangles (EYESY) 168 views, 26 downloads
Random Radii (EYESY) 1 like, 169 views, 16 downloads
Constellations (EYESY) 1 like, 264 views, 14 downloads
MBTA (EYESY) 21 views
Total (Organelle) 4 patches, 17 likes, 3,176 views, 588 downloads
Total (EYESY) 23 patches, 4 likes, 1,687 views, 146 downloads
Total: 27 patches, 21 likes, 4,863 views, 734 downloads

Posted on

Experiment 11: Constellations

February was a very busy month for me for family reasons, and it’ll likely be that way for a few months. Accordingly, I’m a bit late on my February experiment, and will likely be equally late with my final experiment as well. I have also stuck with programming for the EYESY, as I have kind of been on a roll in terms of coming up with ideas for it.

This month I created twelve programs for the EYESY, each of which displays a different constellation from the zodiac. I’ve named the series Constellations and have uploaded them to patchstorage. Each one works in exactly the same manner, so we’ll only look at the simplest one, Aries. The more complicated programs simply have more points and lines in them with different coordinates and configurations, but are otherwise are identical.

Honestly, one of the most surprising challenges of this experiment way trying to figure out if there’s any consensus for a given constellation. Many of the constellations are fairly standardized, however others are fairly contested in terms of which stars are a part of the constellation. When there were variants to choose from I looked for consensus, but at times also took aesthetics into account. In particular I valued a balance between something that would look enticing and a reasonable number of points.

I printed images of each of the constellations, and traced them onto graph paper using a light box. I then wrote out the coordinates for each point, and then scaled them to fit in a 1280×720 resolution screen, offsetting the coordinates such that the image would be centered. These coordinates then formed the basis of the program.

import os
import pygame
import time
import random
import math

def setup(screen, etc):
    pass

def draw(screen, etc):
	linewidth = int (1+(etc.knob4)*10)
 	etc.color_picker_bg(etc.knob5)
	offset=(280*etc.knob1)-140
	scale=5+(140*(etc.knob3))
	r = int (abs (100 * (etc.audio_in[0]/33000)))
	g = int (abs (100 * (etc.audio_in[1]/33000)))
	b = int (abs (100 * (etc.audio_in[2]/33000)))
	if r>50:
		rscale=-5
	else:
		rscale=5
	if g>50:
		gscale=-5
	else:
		gscale=5
	if b>50:
		bscale=-5
	else:
		bscale=5
	j=int (1+(8*etc.knob2))
	for i in range(j):
		AX=int (offset+45+(scale*(etc.audio_in[(i*8)]/33000)))
		AY=int (offset+45+(scale*(etc.audio_in[(i*8)+1]/33000)))
		BX=int (offset+885+(scale*(etc.audio_in[(i*8)+2]/33000)))
		BY=int (offset+325+(scale*(etc.audio_in[(i*8)+3]/33000)))
		CX=int (offset+1165+(scale*(etc.audio_in[(i*8)+4]/33000)))
		CY=int (offset+535+(scale*(etc.audio_in[(i*8)+5]/33000)))
		DX=int (offset+1235+(scale*(etc.audio_in[(i*8)+6]/33000)))
		DY=int (offset+675+(scale*(etc.audio_in[(i*8)+7]/33000)))
		r = r+rscale
		g = g+gscale
		b = b+bscale
		thecolor=pygame.Color(r,g,b)
		pygame.draw.line(screen, thecolor, (AX,AY), (BX, BY), linewidth)
		pygame.draw.line(screen, thecolor, (BX,BY), (CX, CY), linewidth)
		pygame.draw.line(screen, thecolor, (CX,CY), (DX, DY), linewidth)

In these programs knob 1 is used to offset the image. Since only one offset is used, rotating the knob moves the image on a diagonal moving from upper left to lower right. The second knob is used to control the number of superimposed versions of the given constellation. The scale of how much the image can vary is controlled by knob 3. Knob 4 controls the line width, and the final knob controls the background color.

The new element in terms of programing is a for statement. Namely, I use for i in range (j) to create several superimposed versions of the same constellation. As previously stated, the amount of these is controlled by knob 2, using the code j=int (1+(8*etc.knob2)). This allows for anywhere from 1 to 8 superimposed images.

Inside this loop, each point is offset and scaled in relationship to audio data. We can see for any given point the value is added to the offset. Then the scale value is multiplied by data from etc.audio_in. Using different values within this array allows for each point in the constellation to react differently. Using the variable i within the array also allows for differences between the points in each of the superimposed versions. The variable scale is always set to be at least 5, allowing for some amount of wiggle given all circumstances.

Originally I had used data from etc.audio_in inside the loop to set the color of the lines. This resulted in drastically different colors for each of the superimposed constellations in a given frame. I decided to tone this down a bit, by using etc.audio_in data before the loop started allowing each version of the constellation within a given frame to be largely the same color. That being said, to create some visual interest, I use rscale, gscale, and bscale to move the color in a direction for each superimposed version. Since the maximum amount of superimposed images is 8, I used the value 5 to increment the red, green, and blue values of the color. When the original red, green, or blue value was less than 50 I used 5, which moves the value up in value. When the original red, green, or blue value was more than 50 I used -5, which moves the value down in value. The program chooses between 5 and -5 using if :else statements.

The music used in the example videos are algorithms that will be used to generate accompaniment for a third of my next major studio album. These algorithms grew directly out of my work on these experiments. I did add one little bit of code the these puredata algorithms however. Since I have 6 musical examples, but 12 EYESY patches, I added a bit of code that randomly chooses between 1 of 2 EYESY patches and sends out a program (patch) change to the EYESY on MIDI channel 16 at the beginning of each phrase.

While I may not use these algorithms for the videos for the next studio album, I will likely use them in live performances. I plan on doing a related set of EYESY programs for my final experiment next month.

Experiment 11A: Aries & Taurus:

Experiment 11B: Gemini & Cancer:

Experiment 11C: Leo & Virgo:

Experiment 11D: Libra & Scorpio:

Experiment 11E: Sagittarius & Capricorn:

Experiment 11F: Aquarius & Pisces:

Posted on

Experiment 10: Five EYESY Algorithms

I kind of hit a wall of the Organelle. I feel like in order to advance my skills I have a bit of a hurdle between where my programming skills are at, and where they would need to be to do something more advanced that the recent experiments I have completed. Accordingly for this month I decided to shift my focus to the EYESY. Last month I made significant progress in understanding Python programming for the EYESY, and that allowed me to come up with five ideas for algorithms in short order. The music for all five mini-experiments comes from PureData algorithms I will be using for my next major album. All five of these algorithms are somewhat derived from my work on my last album.

The two realizations that allowed me to make significant progress on EYESY programming is that Python is super picky about spaces, tabs, and indentations, and that while the EYESY usually gives little to no feedback when a program has an error in it, you can use an online Python compiler to help figure out where your error is (I had mentioned the latter in last month’s experiment). Individuals who have a decent amount of programming experience may scoff at the simplicity of the programs that follow, but for me it is a decent starting place, and it is also satisfying to me to see how such simple algorithms can generate such gratifying results.

Random Lines is a patch I wrote that draws 96 lines. In order to do this in an automated fashion, I have to use a loop, in this case I use for i in range(96):. The five lines that follow are all executed in this loop. Before the loop commences, we choose the color using knob 4 and the background color using knob 5. I use knob 3 to set the line width, but I scale it by multiplying the knob’s value, which will be between 0 and 1, by 10, and adding 1, as line widths cannot have a value of 0. I also have to cast the value as an integer. I set an x offset value using knob 1. Multiplying by 640 and then subtracting 320 will yield a result between -320 and 320. Likewise, a y offset value is set using knob 2, and the scaling results in a value between -180 and 180.

import os
import pygame
import time
import random
import math

def setup(screen, etc):
    pass

def draw(screen, etc):
	color = etc.color_picker(etc.knob4)
	linewidth = int (1+ (etc.knob3)*10)
 	etc.color_picker_bg(etc.knob5)
	xoffset=(640*etc.knob1)-320
	yoffset=(360*etc.knob2)-180
   	for i in range(96):
		x1 = int (640 + (xoffset+(etc.audio_in[i])/50))
		y1 = int (360 + (yoffset+(etc.audio_in[(i+1)])/90))
		x2 = int (640 + (xoffset+(etc.audio_in[(i+2)])/50))
		y2 = int (360 + (yoffset+(etc.audio_in[(i+3)])/90))
		pygame.draw.line(screen, color, (x1,y1), (x2, y2), linewidth)

Within the loop, I set two coordinates. The EYESY keeps track of the last hundred samples using etc.audio_in[]. Since these values use sixteen bit sound, and sound has peaks (represented by positive numbers) and valleys (represented by negative numbers), these values range between -32,768 and 32,787. I scale these values by dividing by 50 for x coordinates. This will scale the values to somewhere between -655 and 655. For y coordinates I divide by 90, which yields values between -364 and 364.

In both cases, I add these values to the corresponding offset value, and add the value that would normally, without the offsets, place the point in the middle of the screen, namely 640 (X) and 360 (Y). A negative value for the xoffset or the scaled etc.audio_in value would move that point to the left, while a positive value would move it to the right. Likewise, a negative value for the yoffset or the scale etc.audio_in value would move the point up, while a positive value would move it down.

Since subsequent index numbers are used for each coordinate (that is i, i+1, i+2, and i+3), this results in a bunch of interconnected lines. For instance when i=0, the end point of the drawn line (X2, Y2) would become the starting point when i=2. Thus, the lines are not fully random, as they are all interconnected, yielding a tangled mass of lines.

Random Concentric Circles uses a similar methodology. Again, knob five is use to control the background color, while knobs 1 and 2 are again scaled to provide an X and Y offset. The line width is shifted to knob 4. For this algorithm the loop happens 94 times. The X and Y value for the center of the circles is determined the same way as was done in Random Lines. However, we now need a radius and we need a color for each circle. 

import os
import pygame
import time
import random
import math

def setup(screen, etc):
    pass

def draw(screen, etc):
	linewidth = int (1+(etc.knob4)*9)
 	etc.color_picker_bg(etc.knob5)
	xoffset=(640*etc.knob1)-320
	yoffset=(360*etc.knob2)-180
	for i in range(94):
		x = int (640 + xoffset+(etc.audio_in[i])/50)
		y = int (360 + yoffset+(etc.audio_in[(i+1)])/90)
		radius = int (11+(abs (etc.knob3 * (etc.audio_in[(i+2)])/90)))
		r = int (abs (100 * (etc.audio_in[(i+3)]/33000)))
		g = int (abs (100 * (etc.audio_in[(i+4)]/33000)))
		b = int (abs (100 * (etc.audio_in[(i+5)]/33000)))
		thecolor=pygame.Color(r,g,b)
		pygame.draw.circle(screen, thecolor, (x,y), radius, linewidth)

We have knob 3 available to help control the radius of the circle. Here I multiply knob 3 by a scaled version of etc.audio_in[(i+2)]. I scale it by dividing by 90 so that the largest possible circle will mostly fit on the screen if it is centered in the screen. Notice that when we multiply knob 3 by etc.audio_in, there’s a 50% chance that the result will be a negative number. Negative values for radii don’t make any sense, so I take the absolute value of this outcome using abs. I also add this value to 11, as a radius of 0 makes no sense, and a radius of less than 10, as having a line width that is larger than the radius will cause an error.

For this algorithm I take a set forward by giving each circle its own color. In order to do this I have to set the value for red, green, and blue separately, and then combine them together using pygame.Color(). For each of the three values (red, green, and blue) I divide a value of etc. audio_in by 33000, which will yield a value between 0 and 1 (more or less), and then multiply this by 100. I could have done the same thing by simply dividing etc.audio_in by 330, however, at the time this process made the most sense to me. Again, this process could result in a negative number and / or a fractional value, so I cast the result as an integer after getting its absolute value.

Colored Rectangles has a different structure than the previous two examples. Rather than have all the objects cluster around a center point I wanted to create an algorithm that spaces all of the objects out evenly throughout the screen in a grid like pattern. I do this using an eight by eight grid of 64 rectangles. I accomplish the spacing using modulus mathematics as well as integer division. The X value is obtained by multiplying 160 times i%8. In a similar vein, the Y values is set to 90 times i//8. Integer division in Python is accomplished through the use of two slashes. Using this operator will return the integer value of a division problem, omitting the fractional portion. Both the X and the Y values have an additional offset value. The X is offset by (i//8)*(80*etc.knob1), so this offset increases as knob 1 is turned up, with a maximum offset of 80 pixels per row. The value i//8 essentially multiplies that offset by the row number. That is the rows shift further towards the right.

import os
import pygame
import time
import random
import math

def setup(screen, etc):
    pass

def draw(screen, etc):
 	etc.color_picker_bg(etc.knob5)
   	for i in range(64):
		x=(i%8)*160+(i//8)*(80*etc.knob1)
		y=(i//8)*90+(i%8)*(45*etc.knob2)
		thewidth=int (abs (160 * (etc.knob3) * (etc.audio_in[(i)]/33000)))
		theheight=int (abs (90 * (etc.knob4) * (etc.audio_in[(i+1)]/33000)))
		therectangle=pygame.Rect(x,y,thewidth,theheight)
		r = int (abs (100 * (etc.audio_in[(i+2)]/33000)))
		g = int (abs (100 * (etc.audio_in[(i+3)]/33000)))
		b = int (abs (100 * (etc.audio_in[(i+4)]/33000)))
		thecolor=pygame.Color(r,g,b)
		pygame.draw.rect(screen, thecolor, therectangle, 0)

Likewise, the Y offset is determined by (i%8)*(45*etc.knob2). As the value of knob 2 increases, the offset moves towards a maximum value of 45. However, as the columns shift to the right, those offsets compound due to the fact that they are multiplied by (i%8).

A rectangle can be defined in pygame by passing an X value, a Y value, width, and height to pygame.Rect. Thus, the next step is to set the width and height of the rectangle. In both cases, I set the maximum value to 160 (for width) and 90 (for height). However, I scaled them both by multiplying by a knob value (knob 3 for width and knob 4 for height). These values are also scaled by an audio value divided by 33,000. Since negative values are possible from audio values, and negative widths and heights don’t make much sense, I took the absolute value of each. If I were to rewrite this algorithm (perhaps I will), I would set a minimum value for width and height such that widths and heights of 0 were not possible.

I set the color of each rectangle using the same method as I did in Random Concentric Circles. In order to draw the rectangle you pass the screen, the color, the rectangle (as defined by pygame.Rect), as well as the line width to pygame.draw.rect. Using a line width of 0 means that the rectangle will be filled in with color.

Random Rectangles is a combination of Colored Rectangles and Random Lines. Rather than use pygame’s Rect object to draw rectangles on the screen, I use individual lines to draw the rectangles (technically speaking they are quadrilaterals). Knob 4 is used here to set the foreground color, knob 5 is used here to set the background color, knob 3 is used to set the linewidth.

import os
import pygame
import time
import random
import math

def setup(screen, etc):
    pass

def draw(screen, etc):
	color = etc.color_picker(etc.knob4)
 	etc.color_picker_bg(etc.knob5)
	linewidth = int (1+ (etc.knob3)*10)
   	for i in range(64):
		x=(i%8)*160+(i//8)*(80*etc.knob1)+(40*(etc.audio_in[(i)]/33000))
		y=(i//8)*90+(i%8)*(45*etc.knob2)+(20*(etc.audio_in[(i+1)]/33000))
		x1=x+160+(40*(etc.audio_in[(i+2)]/33000))
		y1=y+(20*(etc.audio_in[(i+3)]/33000))
		x2=x1+(40*(etc.audio_in[(i+4)]/33000))
		y2=y1+90+(20*(etc.audio_in[(i+5)]/33000))
		x3=x+(40*(etc.audio_in[(i+6)]/33000))
		y3=y+90+(20*(etc.audio_in[(i+7)]/33000))
		pygame.draw.line(screen, color, (x,y), (x1, y1), linewidth)
		pygame.draw.line(screen, color, (x1,y1), (x2, y2), linewidth)
		pygame.draw.line(screen, color, (x2,y2), (x3, y3), linewidth)
		pygame.draw.line(screen, color, (x3,y3), (x, y), linewidth)

Within the loop, I use a similar method of setting the initial X and Y coordinates. That being said, I separate out the use of knobs and the use of audio input. In the case of the X coordinated, I use (i//8)*(80*etc.knob1) to control the amount of x offset for each row, with a maximum offset of 80. The audio input then offsets this value further using (40*etc.audio_in[(i+2)]/33000). This moves the x value by a value of plus or minus 40 (remember that audio values can be negative. Likewise, knob 2 offsets the Y value for every row by a maximum of 45, and the audio input further offsets this value by plus or minus 20.

Since it takes four points to define a quadrilateral, we need three more points, which we will call (x1, y1), (x2, y2), and (x3, y3). These are all interrelated. The value of X is used to define X1 and X3, while X2 is based off of X1. Likewise, the value of Y helps define Y1 and Y3, with Y2 being based off of Y1. In the case X1 and X2 (which is based on X1) we add 160 to X, giving a default width, but these values are again scaled by etc.audio_in. Similarly, we add 90 to Y1 and Y3 to determine a default height of the quadrilaterals, but again, all points are further offset by etc.audio_in, resulting in quadrilaterals, rather than rectangles with parallel sides. If I were to revise this algorithm I would likely make each quadrilateral a different color.

Frankly, I was not as pleased with the results of Colored Rectangles and Random Rectangles, so I decided to go back create an algorithm that was an amalgam of Random Lines and Random Concentric Circles, namely Random Radii. This program creates 95 lines, all of which have the same starting point, but different end points. Knob 5 sets the background color, while knob 4 sets the line width. 

import os
import pygame
import time
import random
import math

def setup(screen, etc):
    pass

def draw(screen, etc):
	linewidth = int (1+ (etc.knob4)*10)
 	etc.color_picker_bg(etc.knob5)
	xoffset=(640*etc.knob1)-320
	yoffset=(360*etc.knob2)-180
	X=int (640+xoffset)
	Y=int (360+yoffset)
   	for i in range(95):
		r = int (abs (100 * (etc.audio_in[(i+2)]/33000)))
		g = int (abs (100 * (etc.audio_in[(i+3)]/33000)))
		b = int (abs (100 * (etc.audio_in[(i+4)]/33000)))
		thecolor=pygame.Color(r,g,b)
		x2 = int (640 + (xoffset+etc.knob3*(etc.audio_in[(i)])/50))
		y2 = int (360 + (yoffset+etc.knob3*(etc.audio_in[(i+1)])/90))
		pygame.draw.line(screen, thecolor, (X,Y), (x2, y2), linewidth)

Knob 1 & 2 are used for X and Y offsets (respectively) of the center point. Using (640*etc.knob1)-320 means that the X value will move plus or minus 320. Similarly, (360*etc.knob2)-180 permits the Y value to move up or down by 180. As is the case with Random Concentric Circles, the color of each line is defined by etc.audio_in. Knob 3 is used to scale the end point of each line. In the case of both the X and Y values, we start from the center of the screen (640, 360) add the offset defined by knobs 1 or 2, and then use knob 3 to scale a value derived from etc.audio_in. Since audio values can be positive or negative, these radii shoot outward from the offset center point in all directions.

As suggested earlier, I am very gratified with these results. Despite the simplicity of the Python code, the results are mostly dynamic and compelling (although I am somewhat less than thrilled with Colored Rectangles and Random Rectangles). The user community for the EYESY is much smaller than that of the Organelle. The EYESY user’s forum has only 13% the activity of that of the Organelle. I seem to have inherited being the administrator of the ETC / EYESY Video Synthesizer from Critter&Guitari group on Facebook. Likewise, I am the author of the seven most recent patches for the EYESY on patchstorage. Thus, this month’s experiment sees me shooting to the top to become the EYESY’s most active developer. The start of the semester has been very busy for me. I am somewhat doubting that I will be coming up with an Organelle patch next month, but I equally suspect that I will continue to develop more algorithms of the EYESY.

Posted on

Experiment 9: Wavetable Sampler

This month’s experiment is a considerable step forward on three fronts: Organelle programming, EYESY programming, and computer assisted composition. In terms of Organelle programming, rather than taking a pre-existing algorithm and altering it (or hacking it) to do something different, I decided to create a patch from scratch. I first created it in PureData, and then reprogrammed it to work specifically with the Organelle. Creating it in PureData first meant that I used horizontal sliders to represent the knobs, and that I sent the output to the DAC (digital to analog converter). When coding for the Organelle, you use a throw~ object to output the audio.

The patch I wrote, Wavetable Sampler, reimagines a digital sampler, and in doing so, basically reinvents wavetable synthesis. The conventional approach to sampling is to travel through the sound in a linear fashion from beginning to end. The speed at which we travel through the sound determines both its length and its pitch, that is faster translates to shorter and higher pitched, while slower means longer and lower pitched.

I wanted to try using an LFO (low frequency oscillator) to control where we are in a given sample. Using this technique the sound would go back and forth between two points in the sample continuously. In my programming I quickly learned that two parameters are strongly linked, namely the frequency of this oscillator and the amplitude of the oscillator, which becomes the distance travelled in the sample. If you want the sample to be played at a normal speed, that is we recognize the original sample, those two values need to be proportional. To describe this simply, a low frequency would require the sample to travel farther while a higher frequency would need a small amount of space. Thus, we see the object expr (44100 / ($f1)), with the number 44,100 being the sample rate of the Organelle. Dividing the sample rate by the frequency of the oscillator yields the number of samples that make up a cycle of sound at that frequency.

Obviously, a user might want to specifically move at a different rate than what would be normal. However, making that a separate control prevents the user from having to mentally calculate what would be an appropriate sample increment to have the sample play back at normal speed. I also specified that a user will want to control where we are in a much longer sample. For instance, the sample I am using with this instrument is quite long. It is an electronic cello improvisation I did recently that lasts over four minutes.

The sound I got out of this instrument was largely what I expected. However, there was one aspect that stood out more than I thought it would. I am using a sine wave oscillator in the patch. This means that the sound travels quickly during the rise and fall portion of the waveform, but as it approaches the peak and trough of the waveform it slows down quite dramatically. At low frequencies this results in extreme pitch changes. I could easily have solved this issue by switching to a triangle waveform, as speed would be constant using such a waveform. However, I decided that the oddness of these pitch changes were the feature of the patch, and not the bug.

While I intended the instrument to be used at low frequencies, I found that it was actually far more practical and conventionally useful at audio frequencies. Human hearing starts around 20Hz, which means if you were able to clap your hands 20 times in a second you would begin to hear it as a very low pitch rather than a series of individual claps. One peculiarity of sound synthesis is that if you repeat a series of samples, no mater how random they may be, at a frequency that lies within human hearing, you will hear it as a pitch that has that frequency. The timbre between two different sets of samples at the same frequency may vary greatly, but we will hear them as being, more or less, the same pitch.

Thus, as we move the frequency of the oscillator up into the audio range, it turns into somewhat of a wavetable synthesizer. While wavetable synthesis was created in 1958, it didn’t really exist in its full form until 1979. At this point in the history of synthesis it was an alternative to FM synthesis, which could offer robust sound possibilities but was very difficult to program, and digital sampling, which could recreate any sound that could be recorded but was extremely expensive due to the cost of memory. In this sense wavetable synthesis is a data saving tool. If you imagine a ten second recording of a piano key being struck and held, the timbre of that sound changes dramatically over those ten seconds, but ten seconds of sampling time in 1980 was very expensive. Imagine if instead we can digitize individual waveforms at five different locations in the ten second sample, we can then gradually interpolate between those five waveforms to create a believable (in 1980) approximation of the original sample. That being said, wavetable synthesis also created a rich, interesting approach to synthesizing new sounds such that the technique is still somewhat commonly used today.

When we move the oscillator for Wavetable Sampler into the audio range, we are essentially creating a wavetable. The parameter that effects how far the oscillator travels through the sample creates a very interesting phenomenon at audio rates. When that value is very low, the sample values vary very little. This results in waveforms that approach a sine wave in their simplicity and spectrum. As this value increases more values are included, which may differ greatly from each other. This translates into adding harmonics into the spectrum. Which harmonics are added are dependent up the wavetable, or snippet of a sample, in question. However, as we turn up the value, it tends to add harmonics from lower ones to higher ones. At extreme values, in this case ten times a normal sample increment, the pitch of the fundamental frequency starts to be over taken by the predominant frequencies in that wavetable’s spectrum. One final element of interest with the construction of the instrument in relation to wavetable synthesis is related to the use of a sine wave for the oscillator. Since the rate of change speeds up during the rise and fall portion of the waveform and slows down near the peak and the valley of the wave, that means there are portions of the waveform that rich in change while other portions where the rate of change is slow.

Since the value that the oscillator travels seems to be analogous to increasing the harmonic spectrum, I decided to put that on knob four, as that is the knob I have been controlling via breath pressure with the WARBL. On the Organelle I set knob one to set the index of where we are in the four minute plus sample. The frequency of the oscillator is set by the key that is played, but I use the second knob as a multiplier of this value. This knob is scaled from .001, which will yield a true low frequency oscillator, to 1, which will be at the pitch played (.5 will be down an octave, .25 will be down two octaves, etc.). As stated earlier, the fourth knob is used to modify the amplitude of the oscillator, affecting the range of samples that will be cycled through. This left the third knob unused, so I decided to use that as a decay setting.

The PureData patch that was used to generate the accompaniment for this experiment was based upon the patch created for last month’s experiment. As a reminder, this algorithm randomly chooses between one of four musical meters, 4/4, 3/4, 7/8, and 5/8, at every new phrase. I altered this algorithm to fit a plan I have for six of the tracks on my next studio album, which will likely take three or four years to complete. Rather than randomly selecting them, I define an array of numbers that represent the meters that should be used in the order that they appear. At every phrase change I then move to the next value in the array, allowing the meters to change in a predetermined fashion.

I put the piece of magic that allows this to happen in pd phrasechange. The inlet to this subroutine goes to a sel statement that contains the numbers of new phrase numbers expressed in sixteenth notes. When those values are reached a counter is incremented, a value from the table meter is read, which is sent to the variable currentmeter and the phrase is reset. This subroutine has four outlets. The first starts a blinking light that indicates that the piece is 1/3 finished, the second outlet starts a blinking light that starts when the piece is 2/3 of the way finished. The third outlet starts a blinking light that indicates the piece is on its final phrase. The fourth outlet stops the algorithm, bringing a piece to a close. Those blinking lights are on the right hand side of the screen, located near the buttons that indicate the current meter and the current beat. A performer can then, with some practice watch the computer screen to see what the current meter is, what the current beat is, and to have an idea of where you are in form of the piece.

This month I created my first program for the EYESY, Basic Circles. The EYESY manual includes a very simple example of a program. However, it is too simple it just displays a circle. The circle doesn’t move, none of the knobs change anything, and the circle isn’t even centered. With very little work I was able to center the circle, and change it so that the size of the circle was controlled by the volume of the audio. Likewise, I was able to get knob four to control the color of the circle, and the fifth knob to control the background color.

However, I wanted to create a program that used all five knobs on the EYESY. I quickly came up with the idea of using knob two to control the horizontal positioning, and the third knob to control the vertical positioning. I still had one knob left, and only a simple circle in the middle of the screen to show for it. I decided to add a second circle, that was half the size of the first one. I used knob five to set the color for this second circle, although oddly it does not result in the same color as the background. Yet, this still was not quite visually satisfying, so I set knob one to set an offset from the larger circle. Accordingly, when knob one is in the center, the small circle is centered within the larger one. As you turn the knob to the left the small circle moves to the upper left quadrant of the screen. As you turn the knob to the right the smaller circle moves towards the lower right quadrant. This is simple, but offers just enough visual interest to be tolerable.

import os
import pygame
import time
import random
import math

def setup(screen, etc):
    pass

def draw(screen, etc):
	size = (int (abs (etc.audio_in[0])/100))
	size2 = (int (size/2))
	position = (640, 360)
	color = etc.color_picker(etc.knob4)
	color2 = etc.color_picker(etc.knob5)
	X=(int (320+(640*etc.knob2)))
	X2=(int (X+160-(310*etc.knob1)))
	Y=(int (180+(360*etc.knob3)))
	Y2=(int (Y+90-(180*etc.knob1)))
	etc.color_picker_bg(etc.knob5)  
	pygame.draw.circle(screen, color, (X,Y), size, 0)
	pygame.draw.circle(screen, color2, (X2,Y2), size2, 0)

While the program, listed above, is very simple, it was my first time programming in Python. Furthermore, targeting the EYESY is not the simplest thing to do. You have plug a wireless USB adapter into the EYESY, connect to the EYESY via a web browser, upload your program as a zip file, unzip the file, and then delete the zip file. You then have to restart the video on the EYESY to see if the patch works. If there is an error in your code, the program won’t load, which means you cannot trouble shoot it, you just have to look through your code line by line and figure it out. Although, I learned to use an online Python compiler to check for basic errors. If you have a minor error in your code the EYESY will sometimes load the program and display a simple error message onscreen, which will allow you to at least figure where the error is.

I’m very pleased with the backing track, and given that it is my first program for the EYESY, with the visuals. I’m not super pleased with the audio from the Organelle. Some of this is due to my playing. For this experiment I used a very limited set of pitches in my improvisation, which made the fingering easier than it has been in other experiments. Also, I printed out a fingering chart and kept it in view as I played. Part of it is due to my lack of rhythmic accuracy. I am still getting used to watching the screen in PureData to see what meter I am in and what the current beat is. I’m sure I’ll get the hang of it with some practice.

One fair question to ask is do I continue to use the WARBL to control the Organelle if I consistently find it so challenging? The simple answer is that consider a wind controller to be the true test of the expressiveness of a digital musical instrument. I should be able to make minute changes to the sound by slight changes in breath pressure. After working with the Organelle for nine months, I can say that it fails this test. The knobs on the Organelle seem to quantize at a low resolution. As you turn a knob you are changing the resistance in a circuit. The resulting current is then quantized, that is its absolute value is rounded to the nearest digital value. I have a feeling that the knobs on the Organelle quantize to seven bits in order to directly correspond to MIDI, which is largely a seven bit protocol. Seven bits of data only allow for 128 possible values. Thus, we hear discrete changes rather than continual ones as we rotate a knob. For some reason I find this short coming is amplified rather than softened when you control the Organelle with a wind controller. At some point I should do a comparison where I control a PureData patch on my laptop using the WARBL without using the Organelle.

I recorded this experiment using multichannel recording, and I discovered something else that is disappointing about the Organelle. I found that there was enough background noise coming from the Organelle that I had to use a noise gate to clean up the audio a bit. In fact, I had to set the threshold at around -35 dB to get rid of the noise. This is actually pretty loud. The Volca Keys also requires a noise gate, but a lower threshold of -50 or -45 dB usually does the trick with it.

Perhaps this noise is due to a slight ground loop, a small short in the cable, RF interference, or some other problem that does not originate in the Organelle, but it doesn’t bode well. Next month I may try another FM or additive instrument. I do certainly have a good head start on the EYESY patch for next month.

Posted on

Experiment 8: Bass Harmonica

For this month’s experiment I created a sample player that triggers bass harmonica samples. I based the patch off of Lo-Fi Sitar by a user called woiperdinger. This patch was, in turn, based off of Lo-Fi Piano by Henr!k. According to this user, this was based upon a patch called Piano48 by Critter and Guitari.

The number 48 in the title refers to the number of keys / samples in the patch. Accordingly, my patch has the potential for 48 different notes, although only 24 notes are actually implemented. This is due to the fact that the bass harmonica I used to create the sample set only features 24 pitches. I recorded the samples using a pencil condenser microphone. I pitch corrected each note (my bass harmonica is fairly out of tune at this point), and I EQed each note to balance the volumes a bit. Initially I had problems as I had recorded the samples at a higher sample rate (48kHz) than the Organelle operates at (44.1kHz). This resulted in the pitch being higher than planned, but it was easily fixed by adjusting the sample rate on each sample. I had planned on recording the remaining notes using my Hohner Chromonica 64, but I ran out of steam. Perhaps I will expand the sample set in a future release.

The way this patch works is that every single note has its own sample. Furthermore, each note is treated as its own voice, such that my 24 note bass harmonica patch allows all 24 notes to be played simultaneously. The advantage of having each note have its own sample is that each note will sound different, allowing the instrument to sound more naturalistic. For instance the low D# in my sample set is really buzzy sounding, because that note buzzes on my instrument. Occasionally hitting a note with a different tone color makes it sound more realistic. Furthermore, none of the samples have to be rescaled to create other pitches. Rescaling a sample either stretches the time out (for a lower pitch) or squashes the time (for a higher pitch), again creating a lack of realism.

The image above looks like 48 individual subroutines, but it is actually 48 instantiations of a single abstraction. The abstraction is called sampler-voice, and each instance of this abstraction is passed the file name of the sample along with the key number that triggers the sample. The key numbers are MIDI key numbers, where 60 is middle C, and each number up or down is one chromatic note away (so 61 would be C#).

Inside sampler-voice we see basically two algorithms, one that executes when the abstraction loads (using loadbang), and one that runs while the algorithm is operating. If we look at the loadbang portion of the abstraction, we see that it uses the object soundfiler to load the given sample into an array. This sample is then displayed in the canvas to the left. Soundfiler sends the number of samples to its left outlet. This number is then divided by 44.1 to yield the time in milliseconds.

As previously stated, the balance of the algorithm operates while the program is running. The top part of the algorithm, r notes, r notes_seq, r zero_notes, listens for notes. The object stripnote is being used to isolated the MIDI note number of the given event. This is then passed through a mod (%) 48 object as the instrument itself only has 48 notes. I could have changed this value to 24 if I wanted every sample to recur once every two octaves. The object sel $2 is then use to filter out all notes except the one that should trigger the given sample ($2 means the second value passed to the abstraction). The portion of the algorithm that reads the sample from the array is tabread4~ $1-array.

Knob 1 of the Organelle is used to control the pitch of the instrument (in case you want to transpose the sample). The second knob is used to control both the output level of the reverb as well as the mix between the dry (unprocessed) sound and the wet (reverberated) sound. In Lo-Fi Sitar, these two parameters each had their own knob. I combined them to allow for one additional control. Knob 3 is a decay control that can be used if you don’t want the whole sample to play. The final knob is used to control volume, which is useful when using a wind controller, such as the Warbl, as that can be used to allow the breath control to control the volume of the sample.

The PureData patch that controls the accompaniment is basically the finished version of the patch I’ve been expanding through this grant project. In addition to the previously used meters 4/4, 3/4, and 7/8, I’ve added 5/8. I’d share information about the algorithm itself, but it is just more of the same. Likewise, I haven’t done anything new with the EYESY. I’m hoping next month I’ll have the time to tweak an existing program for EYESY, but I just didn’t have the time to do that this month.

I should have probably kept the algorithm at a slower tempo, as I think the music works better at a slower tempo. The bass harmonica samples sound fairly natural, except for when the Organelle seems to accidentally trigger multiple samples at once. I have a theory that the Warbl uses a MIDI On message with a velocity of 0 to stop notes, which is an acceptable use of a MIDI On message, but that PureData uses it to trigger a note. If this is the case, I should be able to fix it in the next release of the patch.

It certainly sounds like I need to practice my EVI fingering more, but I found the limited pitch range (two octaves) of the sample player made the fingering easier to keep track of. Since you cannot use your embouchure with an EVI, you use range fingerings in order to change range. With the Warbl, your right hand (lower hand) is doing traditional valve fingerings, while your left hand (upper hand) is doing fingerings based upon traditional valve fingerings to control what range you are playing on. Keeping track of how the left hand affects the notes being triggered by the right hand has been my stumbling block in terms of learning EVI fingering. However, a two octave range means you really only need to keep track of four ranges. I found the breath control of the bass harmonica samples to be adequate. I think I’d really have to spend some time calibrating the Warbl and the Organelle to come up with settings that will optimize the use of breath control. Next month I hope to create a more fanciful sample based instrument, and maybe move forward on programming for the EYESY.

Posted on

Sabbatical: Week 11 Update

This week I managed to mix and incorporate the Jetliner String Quartet recording for 737. I also was able to record eight phrases on my electric cello. All in all, I recorded two phrases for each of the following: 737, DC-8, 707, & 747. While I haven’t played cello regularly in nearly 40 years, I find that I am slightly better at it than playing the trombone. I’m still not particularly good at playing the instrument, but if you slap a bunch of effects on it, it does sound nice and spacey.

I’ve also been re-editing the string orchestra samples. One of the first things I did for the Rotate project was to add samples of Musiversal’s Budapest String Orchestra that I had recorded for my previous album. These samples were added right after the backing tracks were recorded. Accordingly, I added a lot of them, and now that the recordings are getting kind of thick, I want to thin out the string orchestra samples so they do not compete as much with the string quartet recordings. I managed to thin out TriStar and 737 in this manner. All in all, it was a decent amount of work accomplished for a week in which I was driving to tech rehearsals in Andover, MA for more than half of the week. It puts me a bit ahead of the game in terms of what I hope to accomplish next week.

Since I’ve been posting teasers related to the next album project over the last couple of updates, I’ll share a bit more. I’m pleased to announce that I have working algorithms for two of the six movements that I plan on recording the backing tracks for this coming summer. At the rate I’m crafting these algorithms, I could be ready to record those backing tracks sometime in early 2024. Regardless, I will start sharing examples from these algorithms in early in the new year.

As I posted a link to the new mix of DC-8 featuring the Jetliner String Quartet record, I’ll repost the string score for the movement. This is the only movement that uses quarter note arpeggiations. I’m also fond of the D# diminished chord over an E pedal at rehearsal C. I think it’s a particularly tasty harmony.

Posted on

Sabbatical: Week 10 Update

I had hoped to post this on Friday, Saturday, or Sunday, but it has been a busy time. The good news is that I got more work done than I had expected to. I mixed and incorporated the string quartet recordings for eight of the nine movements. Some of the movements had multiple usable takes, so in some instances I chose to double (or even in 1 instance, triple) track the string quartet recordings to thicken things up a bit. Ultimately, I was able to add string quartet recordings to TriStar, A300, DC-8, 727, 707, DC-10, DC-9, and 747.

This will be a busy week for me as I am in tech week for a production of A Wrinkle in Time up in Andover. That being said, I expect to be able to complete the final string quartet mix, and to be able to get started recording some electric cello, which will put me a bit ahead of schedule. Since I have little to share this week, I’ll share a bit more about my next album project that I unveiled last week.

My plan is to have the album consist of 18 tracks, which should make a good middle ground between ME7ROPOL17AN 7RANSPOR7A71ON AU74R17Y‘s lots of short tracks approach, and Rotate‘s a few long tracks approach. As is the case with Rotate, the drum machine and synth parts will be generated by algorithms written in PureData. However, there will be three different broad models for these algorithms, so that this forthcoming album will feature more variety. The plan is to record the backing tracks to 6 of the movements during the summer of 2024, another 6 (using different algorithms) during the following summer, and a final 6 movements (using a third set of algorithms) during the summer of 2026.

Since I’ve released the recording of TriStar featuring the string quartet recording, I’ll re-share the score for the quartet for those who want to follow along . . .

Posted on

Experiment 7: Additive Odd / Even

I’m afraid I’m not as pleased with this month’s entry as I had hoped to be. The instrument I developed worked fairly well on the Organelle, but when I used it in combination with a wind controller, it was not nearly as expressive as I had hoped. I had also hoped to use the EYESY with a webcam, but I was not able to get the EYESY to recognize either of my two USB webcams. That being said, I think the instrument I designed is a good starting point for further development.

The instrument I designed, Additive Odd Even, is an eight-voice additive synthesizer. Additive synthesis, as the name implies, is an alternative approach to subtractive synthesis. Subtractive synthesis was the most common approach for the first decades of synthesis, as it requires the fewest / least expensive components in comparison to most other approaches. Subtractive synthesis involves taking periodic waveforms that have rich harmonic content, and using filters to subtract some of that content to create new sounds.

Additive synthesis was theorized by rarely attempted since the beginning of sound synthesis. Technically speaking the early behemoth, the Teleharmonium, used additive synthesis. Likewise, earlier electronic organs often used some variant of additive synthesis. One of the few true early additive synthesizers was the Harmonic Tone Generator. However, this instrument’s creator, James Beauchamp only made two of them.

Regardless, additive synthesis involves adding pure sine tones together to create more complex waveforms. In typical early synthesizers, this was impractical, as it would require numerous expensive oscillators in order to accomplish this approach. As a point of reference, the Harmonic Tone Generator only used six partials.

Additive Odd Even is based upon Polyphonic Additive Synth by user wet-noodle. In my patch, knob one controls the transposition level, allowing the user to raise or lower the pitch chromatically up to two octaves in either direction. The second knob controls the balance of odd versus even partials. When this knob is in the middle, the user will get a sawtooth wave, and when it is turned all the way to the left, a square wave will result. Knob three controls both the attack and release, which are defined in terms of milliseconds, ranging from 0 to 5 seconds. The final knob controls the amount of additive synthesis applied, yielding a multiplication value of 0 to 1. This last knob is the one that is controlled by the amount of breath pressure from the WARBL. Thus, in theory, as more breath pressure is supplied, we should hear more overtones.

This instrument consists only of a main routine (main.pd) and one abstraction (voice.pd). Knob one is controlled in the main routine, while the rest exist in the abstraction. As we can see below, voice.pd contains 20 oscillators, which in turn provide 20 harmonic partials for the sound. We can see this in the way in which the frequency of each successive oscillator is multiplied by integers 1 through 20. A bit below these oscillators, we see that the amplitudes of these oscillators is multiplied by successively smaller values from 1 down to .05. These values roughly correspond to 1/n, where n is the harmonic value. Summing these values together would result in a sawtooth waveform.

We see more multiplication / scaling above these values. Half of them come directly from knob 2, which controls the odd / even mix. These are used to scale only the even numbered partials. Thus, when the second knob is turned all the way to the left, the result is 0, which effectively turns off all the even partials. This results in only the odd partials being passed through, yielding a square waveform. The odd numbered partials are scaled using 1 minus the value from the second knob. Accordingly, when knob 2 is placed in the center position, the balance between the odd and even partials should be the same, yielding a sawtooth wave. Once all but the fundamental is scaled by knobs 2 & 4, they are mixed together, and mixed with the fundamental. Thus, we can see that neither knob 2 nor 4 affects the amplitude of thefundamental partial. This waveform is then scaled by .75 to avoid distortion, and then scaled by the envelope, provided by knob three.

In August I had about one month of data loss. Accordingly, I lost much of the work I did on the PureData file that I used to generate the accompaniment for Experiment 5. Fortunately I had the blog entry for that experiment to help me reconstruct that program. I also added a third meter, 7/8, in addition to the two meters used in Experiment 5 (4/4 and 3/4). Most of the work to add this is adding a bunch of arrays, and continuing the expansion of the algorithm that was already covered in the blog entry for Experiment 5.

That being said, using an asymmetrical meter such as 7/8 creates a challenge for the visual metronome I added in experiment 5. Previously I was able to put a select statement, sel 0 4 8 12 that comes from the counter that tracks the sixteenth notes in a given meter. I could then connect each of the four leftmost outlets of that sel statement to a bang. Thus, each bang would activate in turn when you reach a downbeat (once every 4 sixteenth notes).

However, asymmetrical meters will not allow this to work. As the name suggests, in such meters the beat length is inconsistent. That is there are beats that are longer, and ones that are shorter. The most typical way to count 7/8 is to group the eighth notes with a group of 3 at the beginning, and to have two groups of 2 eighths at the end of the measure. This results in a long first beat (of 3 eighths or 6 sixteenth notes), followed by two short beats (of 2 eighths or 4 sixteenth notes).

Accordingly, I created a new subroutine called pd count, which routes the sixteenth note count within the current measure based upon the current meter. Here we see that the value of currentmeter or a 0 sent by initialize is sent to a select statement that is used to turn on one of three spigots, and shut off the others. The stream is then sent to one of two select statements that identify when downbeats occur. Since both 4/4 an 3/4 use beats that are 4 sixteenth notes long, both of those meters can be sent to the same select statement. The other sel statement, sel 0 6 10, corresponds to 7/8. The second beat does not occur until the sixth sixteenth note, while the final downbeat occurs 4 sixteenth notes later at count 10.

One novel aspect of this subroutine is that it has multiple outlets. Each outlet is fed a bang. Each outlet of the subroutine is sent to a different bang, so the user can see the beats happen in real time. Note that this is next to a horizontal radio button, which displays the current meter. Thus, the user can use this to read both the meter, and which beat number is active.

I had to essentially recreate the code inside pd count inside of pd videoautomation in order to change the value of knob 5 of the EYESY on each downbeat. Here the output from the select statements are sent to messages of 0 through 3, which correspond to beats 1 through 4. These values are then used as indexes to access values stored in the array videobeats.

I did not progress with my work on the EYESY during this experiment, as I had intended to use the EYESY in conjunction with a webcam, but unfortunately I could not get the EYESY to recognize either of my two USB webcams. I did learn that you can send MIDI program changes to the EYESY to select which scene or program to make use of. However, I did not incorporate that knowledge into my work.

One interesting aspect of the EYESY related to program changes is that it runs every program loaded onto the EYESY simultaneously. This allows seamless changes from one algorithm to another in real time. There is no need for a new program to be loaded. This operational aspect of the EYESY requires the programs be written as efficiently as possible, and Critter and Guitari recommends loading no more than 10MB of program files onto the EYESY at any given time so the operation does not slow down.

As stated earlier, I was disappointed in the lack of expression of the Additive Odd Even patch when controlled by the WARBL. Again, I need to practice my EVI fingering. I am not quite use to reading the current meter and beat information off of the PureData screen, but with some practice, I think I can handle it. While the programming changes for adding 7/8 to the program that generates the accompaniment is not much of a conceptual leap from the work I did for Experiment 5, it is a decent musical step forward.

Next month I hope to make a basic sample instrument for the Organelle. I will likely add another meter to the algorithm that generates accompaniment. While I’m not quite sure what I’ll do with the EYESY, I do hope to move forward with my work on it.

Posted on

Experiment 6: Constant Gardener

It has been a busy month for me, so I’m afraid this experiment is not as challenging as it could be. I used the Organelle patch Constant Gardener to process sound from my lap steel guitar. This patch uses a phase vocoder, which allows the user to control speed and pitch of audio independently from each other. While I won’t go into great detail about what a phase vocoder is and how it works, it uses a fast Fourier transform algorithm to analyze an audio signal and to reinterpret it as time-frequency representation.

This process is dependent upon the Fourier analysis. The idea behind Fourier analysis is that complex functions can be represented as a sum of simpler functions. In audio this idea is used to separate complex audio signals into its constituent sine wave components. This idea is central to the concept of additive synthesis, which is based upon the idea that any sound, no matter how complex, can be represented by a number of sine wave elements that can be summed together. When we convert an audio signal to a time-frequency representation we get a three-dimensional analysis of the sound where one dimension is time, one dimension is frequency, and the third dimension is amplitude.

Not only can we use this data to resynthesize a sound, but in doing so, we can treat time and frequency separately. That is we can slow a sound down without making the pitch go lower. Likewise, we could raise the pitch of a sound without making the sound wave shorter.

Back to Constant Gardener. This patch uses knob 1 to control the speed (or time element) of the re-synthesis. Knob 2 controls the pitch of the resynthesis. The third knob controls the balance between the dry audio input to the Organelle with the processed (resynthesized) sound. The final knob controls how much reverb is added to the sound. The aux button (or foot pedal) is used to turn the phase vocoder resynthesis on or off.

The phase vocoder part of the algorithm is sufficiently difficult such that I won’t attempt to go through it here, rather I will go through the reverb portion of the patch. As stated previously, knob four controls the balance between the dry (non-reverberated) and the reverberated sound. Thus value is then sent to the screen as a percentage, and is also sent to the variable reverb-amt using a number from 0 to 1 inclusive.

When the value of reverb-amt is recieved, it is sent to a subroutine called cg-dw. I’m not sure why the author of the patch used that name (perhaps cg stands for constant gardener), but this subroutine basically splits the signal in two, and modifies the value the will be returned out of the left outlet to be the inverse of the value of the right outlet (that is 1 – the reverb amount). Both values are passed through a low pass filter with cutoff frequency of 5 Hz, presumably to smooth out the signal.

The object lop~ 10000 receives its input from a chain that can be traced back to the input of the dry audio coming from the Organelle’s audio input. This object is a low pass filter, which means that the frequencies below the cutoff frequency, in this case 10,000 Hz, to pass through the filter, which in return attenuates the frequencies above the cutoff frequency. More specifically, lop is a one-pole, which means that the amount of attenuation is 6 dB per octave. A reduction of 6 dB effectively is half the power of the original. Thus, if the cutoff frequency of a low pass filter is set to 100 Hz, the power at 200 Hz (doubling a frequency raises the pitch an octave) is half of what it would normally be, and at 400 Hz, the power would be a quarter of what it would normally be.

In analog synthesis a two pole (12 dB / octave reduction) or a four pole (24 dB / octave) filter would be considered more desirable. Thus, a one pole filter can be thought of as a fairly gentle filter. This low pass filter is put in the signal chain to reduce the high frequency content to avoid aliasing. Aliasing is the creation of artifacts when a signal is not sampled frequently enough to faithfully represent a signal. Human beings can hear up to 20,000 Hz, but audio demands at least one positive value and one negative value to represent a sound wave. Thus, CD quality sound uses 44,100 samples per second. The Nyquist frequency, the frequency at which aliasing starts is half the sample rate. In the case of CD quality audio, that would be 22,050 Hz. Thus, our low pass filter reduces these frequencies by more than half.

The signal is then passed to the object hip~ 50. This object is a one-pole high pass filter. This type of filter attenuates the frequencies below the cutoff frequency (in this case 50 Hz). Human hearing goes down to about 20 Hz. Thus, the energy at this frequency would be attenuated by more than half. This filter is inserted into the chain to reduce thumps and low frequency noise.

Finally we get to the reverb subroutine itself. The object that does most of the heavy lifting in this subroutine is rev~ 100 89 3000 20. This is a stereo input, four output reverb unit. Accordingly the first two inlets would be the left and right input. The other four inlets are covered by creation arguments (100 89 3000 20). These four values correspond to: output value, liveness, crossover frequency, and high frequency dampening. The output value is expressed in decibels. When expressed in this manner we can think of a change of 10 dB as doubling or halving the volume of a sound. We often consider the threshold of pain (audio so loud that it is physically painful to us) as starting around 120 dB. Thus, 100 dB, while considered to be loud, is 1/4 as loud as the threshold of pain. The liveness setting is really a feedback level (how much of the reverberated sound is fed back through the algorithm). A setting of 100 would yield reverb that would go on forever, while the setting 80 would give us short reverb. Accordingly, 89 gives us a moderate amount of reverb.

The last two values, cross over frequency and high frequency dampening work somewhat like a low pass filter. In the acoustic world low frequencies reverberate very effectively, while high frequencies tend to be absorbed by the environment. That is why a highly reverberant space like a cave or a cathedral has a dark sound to its reverb. In order to model this phenomenon, most reverb algorithms have an ability to attenuate high frequencies built into them. In this case 3,000 Hz is the frequency at which dampening begins. Here dampening is expressed as a percentage. Thus, a dampening of 0 would mean no dampening occurs, while 100 would mean that all of the frequencies about the crossover frequency are dampened. Accordingly, 20 seems like a moderate value. The outlets from pd reverb are then multiplied by the right outlet of cg-dw, applying the amount of reverb desired, and sent to the right and left outputs using throw~ outL and throw~ outR respectively.

For the EYESY I used the patch Mirror Grid Inverse – Trails. The EYESY’s five knobs are used to control: line width, line spacing, trails fade, foreground color, and background color. EYESY programming is accomplished in the language Python, and utilizes subroutines included in a library designed for creating video games called pygame.

An EYESY program is typically in four parts. The first part is where Python libraries are imported (pygame must be imported in any EYESY program). This particular program imports: os, pygame, math, and time. The second part is a setup function. This program uses the code def setup(screen, etc): pass to accomplish this. The third part is a draw function, which will be executed once for every frame of video. Accordingly, while this is where most of the magic happens, it should be written to be as lean as possible in order to run smoothly. Finally, the output should be routed to the screen.

In terms of the performance, I occasionally used knob 2 to change the pitch. I left reverb at 100%, and mix around 50% for the duration of the improvisation. I could have used the keyboard of the Organelle to play specific pitched versions of the audio input. Next month I hope to tackle additive synthesis, and perhaps use a webcam with the EYESY. Given that I’ve given a basic explanation of the first two parts of an EYESY program, in future months I hope to go through EYESY programs in greater detail.